9538: ABC238 —— B - Pizza
[Creator : ]
Description
We have a circular pizza.
Takahashi will cut this pizza using a sequence $A$ of length $N$, according to the following procedure.
- First, make a cut from the center in the $12$ o'clock direction.
- Next, do $N$ operations. The $i$-th operation is as follows.
- Rotate the pizza $A_i$ degrees clockwise.
- Then, make a cut from the center in the $12$ o'clock direction.
For example, if $A=(90,180,45,195)$, the procedure cuts the pizza as follows.
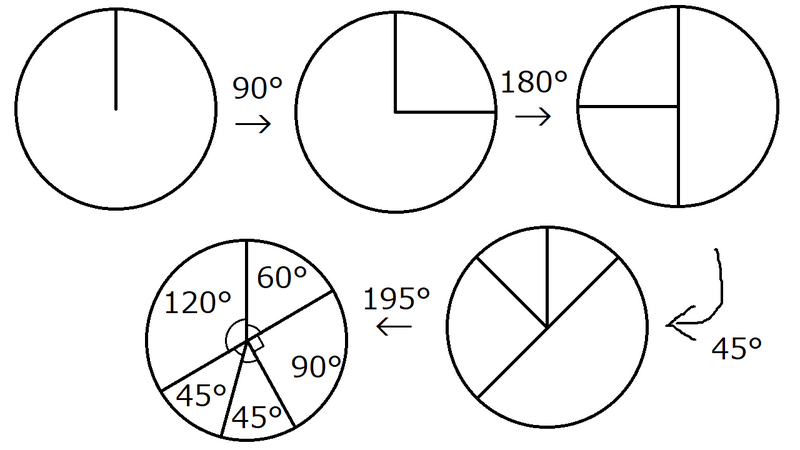
Find the center angle of the largest pizza after the procedure.
Takahashi will cut this pizza using a sequence $A$ of length $N$, according to the following procedure.
- First, make a cut from the center in the $12$ o'clock direction.
- Next, do $N$ operations. The $i$-th operation is as follows.
- Rotate the pizza $A_i$ degrees clockwise.
- Then, make a cut from the center in the $12$ o'clock direction.
For example, if $A=(90,180,45,195)$, the procedure cuts the pizza as follows.

Find the center angle of the largest pizza after the procedure.
Input
Input is given from Standard Input in the following format:
```
$N$
$A_1$ $A_2$ $\dots$ $A_N$
```
```
$N$
$A_1$ $A_2$ $\dots$ $A_N$
```
Output
Print the answer as an integer.
Constraints
- All values in input are integers.
- $1 \le N \le 359$
- $1 \le A_i \le 359$
- There will be no multiple cuts at the same position.
- $1 \le N \le 359$
- $1 \le A_i \le 359$
- There will be no multiple cuts at the same position.
Sample 1 Input
4
90 180 45 195
Sample 1 Output
120
This input coincides with the example in the Problem Statement.
The center angle of the largest pizza is 120 degrees.
The center angle of the largest pizza is 120 degrees.
Sample 2 Input
1
1
Sample 2 Output
359
Sample 3 Input
10
215 137 320 339 341 41 44 18 241 149
Sample 3 Output
170